 D.J. Johnstone, a professor at the University of Sydney Business School in Sydney, Australia, is re-working the capital asset pricing model in accordance with probability theory. I wrote recently about one recent essay of his, on the “Bayesian understanding” of probability theory. Today, I would like to fill out those thoughts. I will allude to two of his other recent articles as I go.
D.J. Johnstone, a professor at the University of Sydney Business School in Sydney, Australia, is re-working the capital asset pricing model in accordance with probability theory. I wrote recently about one recent essay of his, on the “Bayesian understanding” of probability theory. Today, I would like to fill out those thoughts. I will allude to two of his other recent articles as I go.
Simple versions of CAPM often graph the expected return as a function of beta, thus creating the security market line, as portrayed at the top of this entry.
But, Johnstone says in one recent paper, “The Effect of Information on Certainty and the Cost of Capital,” this is a misleading way of formulating CAPM.
A Logical Circularity
He suggests that we consider that the attribute that makes a portfolio optimally diversified is “not what is in it, but what is paid to obtain each of the assets in it.”
For each risky asset (j) an institution spends a specific portion of its endowment (j = 1, 2,...). The remainder stays in a risk-free asset.
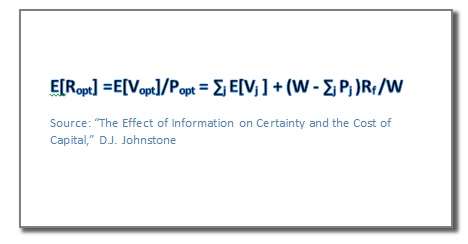
That notion is expressed in the box nearby. In that equation, W is the whole of an endowment, V is the payoff of a risky asset; E refers to expectations and P to price. The significance of the formula is that it illustrates that covariance (beta) is affected by the price of an asset, which in turn is influenced by the expected payoff on that asset.
There is, then, a “logical circularity built into the CAPM equilibrium pricing mechanism,” that is, the expected return depends upon the covariance, the covariance is a function of asset pricing, and the asset pricing depends upon expected return.
The fact that expected payoff helps determine the cost of capital is itself critical to the setting of financial accounting standards. GAAP treats the value of a firm as the expected payoff discounted by a risk-adjusted rate. The above reasoning indicates to Johnstone that the mean payoff plays a big part in both halves of that computation, the expected payoff obviously enough, but the appropriate discount as well.
This allows him to correct what he sees as a common error. As an example of the commonness of this error, he quotes an article the Financial Accounting Standards Board published about itself in 2003, The FASB and the Capital Markets, by John Foster. Foster wrote, “More information always equates to less uncertainty, and … people pay more for certainty. In the context of financial information, the end result is that better disclosure results in a lower cost of capital.”
Improving Eyesight
Johnstone thinks that too optimistic, akin to the claim that improving one’s eyesight will make the world more beautiful. Indeed, in this and another recent paper, “Information, Uncertainty, and the Cost of Capital in a Mean-Variance Efficient Market,” Johnstone explains that there are both winners and losers when accounting standards improve in terms of the information they provide the investing public.
Intuitively, information that allows investors to discriminate more accurately between forms and other assets will lead to greater costs of capital for some and a lowered cost of capital for others. As we improve our eyesight, likewise, some things become more definitely beautiful, other things more definitely ugly.
Less intuitively, a better ability to discriminate among firms can lead to a “higher risk premium on average across the whole market.”
This isn’t of course a case against getting more information to the investors. It is a case against false optimism, because unjustified optimism is the basis of those prices that will fall as information improves. Those price declines will add to the efficiency of the allocation of resources.



